Electric Dipole - Potential At a Point Due To Electric Dipole.

- An electric dipole consists of two point charges of equal magnitude but of opposite sign and separated by a small distance.

- Consider an electric dipole centered at origin and placed in z – axis as shown in the figure:

- The potential (V) at point P is given as:

- If the distance between the charges (d) is very small as compared to the distance of the point P from the origin i.e.

If r >> d,

r₂ – r₁ ≅ d cosθ ;          r₁ ≅ r₂ = r ;           r₁r₂ ≅ r²

Substituting the values in the above equation, the potential at point P becomes:

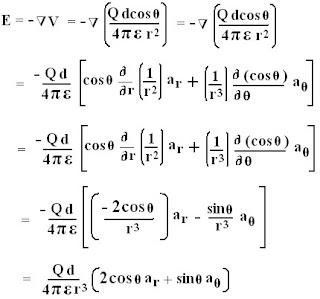
- Electric field intensity (E) is the negative gradient of Electric Potential (V).
Hence,

- The expressions for electric potential (V) and field intensity (E) above are only valid for a dipole centered at the origin and aligned with the z-axis.


- To determine the fields produced by any arbitrary location and alignment, we first need to define a new quantity p, called the Dipole Moment.

p = Q d

- Since the distance d is a vector quantity, the dipole moment p is also a vector quantity.


- Dipole moment p is a measure of the strength of the dipole and indicates its direction.


- Vector d is a directed distance that extends from negative charge (- Q) to positive charge (+ Q). This directed distance vector d thus describes the distance between the dipole charges, as well as the orientation of the charges.

Therefore

d = | d | a_d

Where | d | is the distance between the charges and a_d defines the orientation or direction of the dipole.


- Say a dipole is aligned along z – axis, then directed distance d is given as:

d = | d | a_z

From the above diagram it’s clear that:

a_z . a_r = cosθ

Hence the expression can be written as:

Q d cosθ  =  Q | d | a_z . a_r  =  Q d. a_r  =  p . a_r



- Hence electric potential (V) due to a electric dipole centered at origin and aligned with the z axis is rewritten as:

- The above expression no doubt is applicable for all and any dipole moments p, but is valid for dipoles centered at origin.


- Electric potential (V) at point P with a position vector r due to a dipole centered at a point with position vector r₁ is given as:

ALSO READ: 

- Gauss's Law - Theory.

- Gauss's Law - Application To a Point charge.

- Gauss's Law - Application To An Infinite Line Charge.

- Gauss's Law - Application To An Infinite Sheet Charge.

- Gauss's Law - Application To a Uniformly Charged Sphere.

- Numericals / Solved Examples - Gauss's Law.

- Scalar Electric Potential / Electrostatic Potential (V).

- Relationship Between Electric Field Intensity (E) and Electrostatic Potential (V).

- Electric Potential Due To a Circular Disk.

- Electric Dipole.

- Numericals / Solved Examples - Electric Potential and Electric Dipole.

- Energy Density In Electrostatic Field / Work Done To Assemble Charges.

- Numericals / Solved Examples - Electrostatic Energy and Energy Density.

- Numericals / Solved Examples - Gauss's law...


- Short Notes/FAQ's

Your suggestions and comments are welcome in this section. If you want to share something or if you have some stuff of your own, please do post them in the comments section.