Electric Field Intensity Due To a Finite Line Charge - Field Theory.


- Consider a line charge with uniform charge density ρL extending from + a to – a along the z- axis.


- Charge element dQ associated with the element dl of the line is

 dQ = ρL dl = ρL dz


Electric Field Intensity Due To a Finite Line Charge - Field Theory.


- From the diagram it’s clear that the Electric field intensity has two components i.e. Eρ and Ez.


- If we move around the line charge and if we vary ρ, while keeping φ and z constant, it is expected that the field would become weaker as ρ increases.


- No element of charge produces a φ component i.e. Eφ is equal to zero.


- Hence Electric field intensity at point P has only two component one along the ρ and the other along the z direction.


- However the contribution due to Ez component by the elements of charge will be canceled because the same are at equal distances above and below the point at which the field is to be determined.


(For Example: If a charge element is present at + 4az and another charge element at - 4az, then the z component due to the two mentioned charge element at the point P will be canceled out on resolving the field.)


- Hence the only field component that exist at point P due to a symmetric line charge element is Eρ.


- Differential electric field intensity(dE) due to a small line charge element is given as:


- In this case r is equivalent to l (small L). Therefore the equation can be written as:


- Consider the triangle OPQ, we have


(Small L) l = (z2 + ρ2)1/2


cos θ = ρ / l      ;     sin θ = z / l


dEρ = |dE| cosθ = dE . (ρ / l)

Similarly we have,

dEz = |dE| sinθ = dE . (z / l)

Since the resultant z component Ez of the field at a point on the ρ axis is zero i.e. ∫dEz = 0


The axial component Eρ of the field at a point P on ρ axis can be obtained by integrating from – a to + a as

∫ dEρ = ∫-a+a dE . (ρ / l)


- For an infinite length of line charge “a → ∞"




- Hence the electric field intensity due to an infinite line charge is given as:



To Download This Page: Click Here


ALSO READ:

- Introduction To Electrostatics.

- Coulomb's law.

- Electric Field Intensity (E).

- Electric Lines Of Forces /Streamlines / Electric Flux (ψ) .

- Electric Flux Density (D).

- Electric Field Intensity Due To a Finite and Infinite Line Charge.

- Electric Field Intensity Due To a Infinite Sheet Charge.

- Electric Field Intensity Due To a Circular Ring Charge.

- Electric Field Intensity Due To a Circular Disk Charge.

- Numericals / Solved Examples - Electric Force and Field Intensity.

- Numericals / Solved Examples - Electric Field Intensity - Line, Surface and Mixed Charge Configuration.

- Short Notes/FAQ's

Your suggestions and comments are welcome in this section. If you want to share something or if you have some stuff of your own, please do post them in the comments section.

Comments

  1. AnonymousOctober 17, 2011 at 6:42 PM

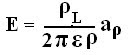
    very nice and helpful

    ReplyDelete
  2. AnonymousJanuary 24, 2012 at 11:49 PM

    hey thank you very much..was helpful to me..

    ReplyDelete
  3. AceFebruary 7, 2012 at 8:40 PM

    Well explained

    ReplyDelete
  4. AnonymousFebruary 8, 2012 at 6:58 PM

    excellent

    ReplyDelete
  5. hatim guitaristFebruary 24, 2012 at 8:06 PM

    simpler than my textbook:-)

    ReplyDelete
  6. UnknownAugust 18, 2012 at 11:33 PM

    excellent understanding of concepts and made easy to understand and revise

    ReplyDelete
  7. UnknownAugust 18, 2012 at 11:34 PM

    excellent understanding of concepts and made easy to revise

    ReplyDelete
  8. UnknownJanuary 2, 2013 at 11:41 PM

    very much useful for better understanding

    ReplyDelete
  9. AnonymousApril 19, 2013 at 10:56 AM

    idk but i think it's wrong..you integrat that withing limits -a to +a intesad of -a/2 to +a/2

    ReplyDelete
  10. UnknownJanuary 20, 2014 at 3:55 PM

    How about let ρ=0, which means the point we care is on the line? When ρ=0, the equation can't work.

    ReplyDelete
  11. AnonymousMay 7, 2014 at 12:04 PM

    very well explained

    ReplyDelete
  12. AnonymousJanuary 23, 2015 at 8:00 PM

    nice

    ReplyDelete

Post a Comment

Popular posts from this blog

Circular Cylindrical Coordinate System (ρ, φ, z) - Field Theory.

Image
A Vector in Cylindrical system is represented as (A ρ , A φ , A z ) or A = A ρ a ρ + A φ a φ + A z a z Where a ρ , a φ and a z are the unit vectors in ρ, φ and z direction respectively. The physical significance of each parameter of cylindrical coordinates:      - The value ρ indicates the distance of the point from the z-axis. It is the radius of the cylinder .      - The value φ, also called the azimuthal angle , indicates the rotation angle around the z-axis. It is basically measured from the x axis in the x-y plane. It is measured anti-clockwise.      - The value z indicates the distance of the point from z-axis. It is the same as in the Cartesian system. In short, it is the height of the cylinder. Range of the variables: It defines the minimum and the maximum value that ρ, φ and z can have in Cartesian system.  0 ≤ ρ ≤ ∞  0 ≤ φ ≤ 2π - ∞ ≤ z ≤ ∞   Figure shows Point P and Unit vectors in Cyli...
Read More »

Electric Field Intensity (E) Due To a Circular Ring Charge - Field Theory.

Image
- Consider a circular ring of radius 'a' which carries a uniform line charge density ρ L as shown in figure. - We need to find out electric field at a point P (0, 0, h) on the z axis (z > 0). - Electric field intensity (E) due to any line charge (ρ L ) in general is given as: In this case, dl = a dφ (Since the differential part dl is a differential arc ) R 2 = a 2 + h 2 Consider the triangle shown in the above figure a a ρ + R = h a z → R = - a a ρ + h a z a R = R / | R | a R = - a a ρ + h a z / ( a 2 + h 2 ) 1/2 Substituting all these values in the above equations, the electric field intensity E becomes: - For every element dl there is a corresponding element diametrically opposite that gives an equal but opposite dE ρ so that the two contributions cancel each other. Hence contribution along a ρ due to symmetry adds up to zero. - Therefore the final electric field intensity at point (0, 0, h) has ...
Read More »

Electric Potential (V) Due To A Uniformly Charged Circular Disc - Field Theory.

Image
- Consider a circular disc of radius ‘a’ which carries a uniform surface charge density ρ s , C /m 2 . - Say the disk lies on x - y plane (or z = 0 plane) with its axis along the z axis as shown in the figure. - We need to find out electric potential (V) due to a circular disk at a point P (0, 0, h) on the z axis (z > 0). - Electric potential (V) at a point due to any surface charge (ρ s ) is given as: - In this case, ds = ρ dρ dφ (Since it’s a disc, the varying terms are radius ρ and angle φ ) R = (ρ 2 + h 2 ) 1/2 - Hence electric potential (V) is given as: - On solving further the equation becomes - As a → 0 , electric potential (V) also tends to zero i.e. V → 0. - Hence the electric potential at point (0, 0, h) is given as: ALSO READ:  - Gauss's Law - Theory. - Gauss's Law - Application To a Point charge. - Gauss's Law - Application To An Infinite Line Charge. - Gauss's ...
Read More »