Differential analysis - Spherical Co-ordinate System - Field Theory.

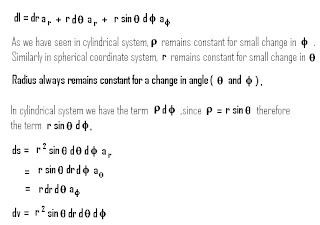
Always remember that for a small change in angle the radius remains constant.

Radius of a cylinder (ρ) is constant for a small change in φ . Similarly the radius of a sphere(r) is constant for a small change in θ.

Therefore ρ always associates dφ , and r always associates dθ.

Differential Length (dl) :

- dl = dra_r + r dθa_θ + ρ dφ a_φ

We know that ρ = rsinθ

Therefore dl = dra_r + r dθa_θ + r sinθdφ a_φ

Differential length for a surface is given as:

- dl = dra_r + r dθa_θ ---( For r - θ plane or φ constant plane)

- dl = r dθa_θ + r sinθdφa_φ---( For θ - φ plane or r constant plane)

- dl = dra_r + r sinθdφ a_φ---( For r - φ plane or θ constant plane)

Differential length for a line parallel to r, θ and φ axis are respectively given as:

- dl = dra_r ---(For a line parallel to r axis)

- dl = r dθa_θ ---( For a line parallel to θ axis)

∫ dl = ∫_o^π rdθ = r (π - 0) = π r

This answer describes the circumference of a semi circle. Hence when θ varies with r and φ constant, the resultant length is the circumference of a semicircle.


- dl = r sinθdφa_φ ---( For a line parallel to φ axis)


Differential Surface (ds):

- ds = r dr dθa_φ

This surface describes a semi circle whose direction is along φ.

∫ ds = ∫_o^r ∫_o^π r drdθ 

= (r²/2) (π - 0) 

= π r²/2

This answer describes the surface area of a semicircle. Hence the surface represents a semicircle.


- ds = r² sinθdθ dφ a_r

This surface describes a hollow sphere or circumference or surface area of a sphere whose direction is along r.

∫ ds = ∫_o^2π ∫_o^π r²sin θ dθdφ 

= - r² (cosπ -cos 0) (2π -0) 

= 4 πr<sup>2

This answer describes the surface area of a sphere. Hence the surface is a sphere with its origin at the origin.</sup>


- ds = rsinθ drdφ a_θ

This describes a circular cone with z-axis as its axis and the origin at its vertex.

Differential Volume (dv):


dv = r² sinθ dr dθ dφ ---(Scalar Quantity)

∫ dv = ∫_o^r ∫_o^π ∫_o^2π r² sinθ drdθdφ 

= - (r³/3) (cosπ - cos 0^o) (2π - 0) 

= 4/3 (π r³)

This answer describes the volume of a sphere.

SOLVED EXAMPLE / NUMERICALS:

Q.2. Express the point P (1, -4, -3) in cylindrical and spherical co-ordinates? SOLUTION/ANSWER


Q.3
a) If V = XZ – XY +YZ, express V in Cylindrical co-ordinate system.

b) If U = X² + 2Y² +3z², express U in Spherical co- ordinates System.   SOLUTION/ANSWER


Q.4 Transform the vector E = (y² – x²) a_x + xyz a_y + (x² – z²) a_x to cylindrical and spherical system?      SOLUTION/ANSWER


Q.5 Express the vector A = ρ (z² + 1)a_ρ - ρz cosφa_φ in Cartesian co-ordinate system?       SOLUTION/ANSWER


Q.6 Express the vector E =2r sinθ cosφa_r + r cosθ cosφa_θ – r sinφa_φ in Cartesian co-ordinate system?SOLUTION/ANSWER


Q.7 Calculate the distance between the following pair of points?

a) (2, 1, 5) and (6, -1, 2)
b) (3, π/2, -1) and (5, 3π/2, 5)
c) (10, π/4, 3π/4) and (5, π/6, 7π/4)     SOLUTION/ANSWER



ALSO READ:

- Introduction To Coordinate System.

- Cartesian Coordinate System / Rectangular Coordinate System (x, y, z).

- Differential Analysis Of Cartesian Coordinate System.

- Circular Cylindrical Coordinate System (ρ, φ, z).

- Differential Analysis Of Cylindrical Coordinate System.


- Spherical Coordinate System ( r, θ , φ).

- Differential Analysis Of Spherical Coordinate System.

- Numericals / Solved Examples - Page 1.

- Numericals / Solved Examples - Page 2.


Your suggestions and comments are welcome in this section. If you want to share something or if you have some stuff of your own, please do post them in the comments section.